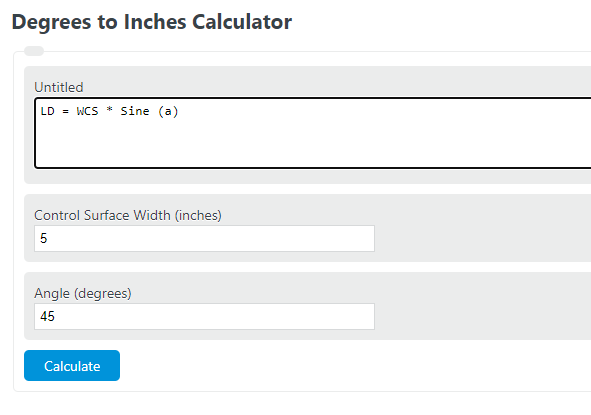
Enter the angle in degrees and the width in inches into the calculator to determine the inches (or mm) of deflection.
- Arc Length Calculator
- MOA Calculator (minute of angle)
- Central Angle Calculator
- MM to Degree Calculator
Degrees to Inches Formula
The following formula is used to convert degrees to inches.
LD = WCS * Sine (a)
- Where LD is the linear deflection (inches)
- WCS is the width of the control surface (inches)
- a is the angle
Degrees to Inches Definition
To convert degrees to inches, you must multiply the width of the control surface in inches by the sine of the angle. This yields the linear deflection in inches.
Degrees to Inches Example
How to convert degrees to inches?
- First, determine the control surface width.
Measure the control surface width in inches.
- Next, determine the angle.
Measure the angle in degrees.
- Finally, calculate the deflection.
Calculate the linear deflection in inches using the formula above.
FAQ
What is the significance of converting degrees to inches in practical applications?Converting degrees to inches is crucial in fields such as engineering, construction, and manufacturing where precise measurements and angles are necessary for the fabrication of parts, assembly of machinery, or construction of structures. It helps in accurately determining the linear displacement or deflection of components when subjected to forces at specific angles.
How does the sine function facilitate the conversion from degrees to inches?The sine function is used in this conversion because it relates the angle of deflection to the linear distance of deflection when considering a circle or arc. By multiplying the width of the control surface (representing the radius in a circular motion) by the sine of the angle, you can calculate the linear deflection perpendicular to the original direction of the control surface. This trigonometric function is essential for converting angular measurements to linear distances in various applications.
Can this formula be used for large angles or should it be limited to small angles?While the formula can theoretically be applied to any angle, it is most accurate for small angles where the linear deflection closely approximates the actual arc length. For larger angles, especially those approaching or exceeding 90 degrees, the approximation may become less accurate due to the properties of the sine function and the increasing difference between the linear deflection and the actual arc length. In such cases, more complex geometric or trigonometric calculations may be required to achieve precise measurements.