Calculate the temperature of a substance given the pressure, volume, and amount of substance through the ideal gas law.
Ideal Gas Law Formula
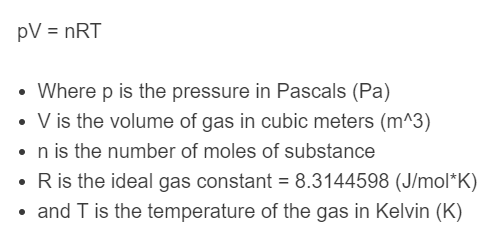
The follow equation is used to define ideal gasses:
pV = nRT
- Where p is the pressure in Pascals (Pa)
- V is the volume of gas in cubic meters (m^3)
- n is the number of moles of substance
- R is the ideal gas constant = 8.3144598 (J/mol*K)
- and T is the temperature of the gas in Kelvin (K)
Ideal Gas Definition
An ideal gas is a form of gas that follows a set of conditions. These conditions mean that the ideal gas can be evaluated in simplified conditions that help with modeling and solving equations. The following is the set of parameters that define an ideal gas
- They obey Newton’s laws of motion
- They don’t interacted except with collisions. I.E. no chemical reactions
- Collisions are elastic
- They are modeled as point particles (take up no space)
- It’s a large amount of substance.
If all of these criteria are met, then this gas can be modeled by the ideal gas law and corresponding equation.
How to calculate ideal gas properties
The properties of a so called ideal gas all follow the equation outlined above. Since the gas constant is already known, only 3 out of 4 properties must be known in order to calculate the missing property. For instance if you know the pressure, volume, and amount of substance you can calculate the temperature. If you know the pressure, temperature, and volume, you can calculate the amount of substance. Simply re-arrange the equation and you are off and running.
The following example will go over the steps to calculating the properties of an ideal gas. In this case specifically, we will solve for pressure.
- First, we must re-arrange the ideal gas equation to solve for pressure. We can simply do this by dividing both sides by volume. The result is the following: p = nrT/V.
- Now that we have pressure solved for in the equation, we need to find or measure the 3 other variables. This would be number of moles, temperature and volume. For this example we will assume 1 mole, 1 degree C temperature, and 1 cubic meter of volume.
- Finally, enter all of that information into the formula and you can find your pressure in pascals.
FAQ
An ideal gas is a form of gas that follows a set of conditions. These conditions mean that the ideal gas can be evaluated in simplified conditions that help with modeling and solving equations. The following is the set of parameters that define an ideal gas
They obey Newton’s laws of motion
They don’t interacted except with collisions. I.E. no chemical reactions
Collisions are elastic
They are modeled as point particles (take up no space)
It’s a large amount of substance.
Related Terms
| ideal gas law calculator |
| ideal gas law formula |
| how to calculate ideal gas law |
| ideal gas law equation |
| gas law equations |
| gas laws formulas |
| general gas equation |
| ideal gas formula |
| ideal gas calculator |
| gas law calculator |
| ideal gas equation units |
| gas equations |
| avogadro’s law calculator |
| gas constant formula |
| the ideal gas equation |
| volume of gas equation |
| universal gas constant formula |
| ideal gas equation calculator |
| universal gas equation |
| gas constant equation |
| pv nrt equation |
| gay lussac’s gas law equation |
| how to find molar mass of an unknown gas |
| equation of ideal gas |
| ideal gas law calculator for volume |
| all gas law formulas |
| molar gas equation |
| moles of gas equation |
| ideal gas equation with density |
| ideal gas density calculator |
| general gas law formula |
| how to find r in ideal gas law |
| density of ideal gas formula |
| general gas law equation |
| ideal gas law calculator density |
| boyle’s gas law equation |
| write the ideal gas equation |
| ideal gas equation formula |
| ideal gas law calculator for moles |
| ideal gas law calc |
| ideal gas equation example |
| formula of gas constant |
| ideal gas equation in terms of density |
| formula of universal gas constant |
| ideal gas equation for one mole of gas |
| charles gas law equation |
| how to find the gas constant |
| ideal gas law solver |
| units of ideal gas equation |
| ideal law formula |
| gas laws definition and formulas |
| avogadro’s gas law equation |
| perfect gas law equation |
| how to find ideal gas constant |
| volume pressure temperature formula |
| pressure of gas equation |
| ideal gas law equation units |
| ideal gas law calculator for pressure |
| gas laws and formulas |
| formula for gas constant |
| ideal gas constant calculator |
| ideal gas density formula |
| formula of ideal gas |
| ideal gas formula units |
| ideal gas equation volume units |
| all gas law equations |
| gas equation units |
| ideal gas law density formula |
| gas laws and equations |
| ideal gas law definition and formula |
| ideal gas equation for n mole of gas |
| van der waals equation real gases |
| equation of gas law |
| ideal gas law molecular weight |
| ideal gas volume calculator |
| general gas law calculator |
| how to calculate universal gas constant |
| the general gas equation |
| ideal gas law formula and examples |
| chemistry gas laws formulas |
| ideal gas molecular weight |
| ideal gas law formula for temperature |
| general gas equation formula |
| mixed gas law formula |
| ideal gas law finding temperature |
| volume temperature pressure equation |
| gas law solver |
| ideal gas law equation example |
| how to find volume in ideal gas law |
| ideal gas law calculator mass |
| how to find density using ideal gas law |
| real gas law calculator |
| ideal gas law conversions |
| ideal gas law formula examples |
| how to find moles using ideal gas law |
| all ideal gas law equations |
| ideal gas law formula for pressure |
| pressure of an ideal gas formula |
| volume formula gas |
| how to find density from ideal gas law |
| how to calculate ideal gas constant |
| pv nrt calculator online |
| formula for universal gas constant |
| ideal gas law calculator with steps |
| gas laws and their formulas |
| universal gas law equation |
| universal gas constant calculator |
| equation of an ideal gas |
| how to find pressure using ideal gas law |
| ideal gas law density equation |
| pressure of ideal gas formula |
| different gas laws and their formula |
| finding density using ideal gas law |
| ideal gas equation graph |
| ideal gas law mathematical equation |
| law of gases formula |
| evaluation of the gas law constant |
| ideal law equation |
| ideal gas equation for density |
| ideal gas law formula for moles |
| ideal gas solver |
| 6 gas laws and their formulas |
| avogadro’s law equation calculator |
| write the equation for the ideal gas law |
| ideal gas pressure calculator |
| different forms of ideal gas equation |
| how to find temperature using ideal gas law |
| perfect gas law calculator |
| n in ideal gas equation |
| corrected ideal gas equation |
| gas law equation calculator |
| ideal gas law all formulas |
| molecular weight ideal gas law |
| ideal gas equation with units |
| ideal gas equation moles |
| ideal gas law to find volume |
| avogadro law ideal gas equation |
| gas constant r formula |
| rearranging the ideal gas equation |
| gas pressure volume calculator |
| ideal gas law derived formulas |
| the correct form of the equation for the ideal gas law is |
| how to calculate molar mass of unknown gas |
| gas law equations pdf |
| ideal law calculator |
| pressure of a gas equation |
| state the general gas equation |
| the ideal gas equation is expressed as |
| ideal gas law to find temperature |
| the ideal gas law calculator |
| how can the ideal gas law be used to determine the molecular mass of a liquid |
| equation for general gas law |
| ideal gas law formula density |