Enter the standard angle into the reference angle calculator. The calculator will evaluate and display the reference angle. This calculator can also evaluate either of the angles in radians.
Reference Angle Formula
The reference angle for any angle can be calculated through the following formulas after first reducing the angle to a coterminal (standard-position) angle in the interval 0° ≤ θ < 360° (or 0 ≤ θ < 2π).
For angles that lie exactly on an axis (0°, 90°, 180°, 270°), the reference angle is 0° or 90° accordingly.
First Quadrant Formula: Reference Angle = Standard Angle
Second Quadrant Formula: Reference Angle = 180° − Standard Angle
Third Quadrant Formula: Reference Angle = Standard Angle − 180°
Fourth Quadrant Formula: Reference Angle = 360° − Standard Angle
In these formulas, the reference angle is also known as the related acute angle. The standard angle is sometimes referred to as the original angle.
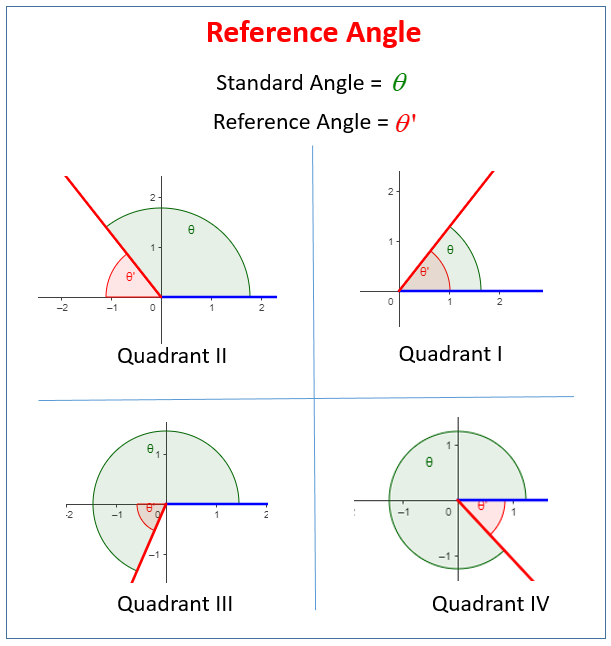
Common Reference Angles
The following list outlines the most common reference angle conversions:
- 30° (π/6), 150° (5π/6), 210° (7π/6), 330° (11π/6) → reference angle 30° (π/6)
- 45° (π/4), 135° (3π/4), 225° (5π/4), 315° (7π/4) → reference angle 45° (π/4)
- 60° (π/3), 120° (2π/3), 240° (4π/3), 300° (5π/3) → reference angle 60° (π/3)
- 0° (0), 180° (π), 360° (2π) → reference angle 0° (0)
- 90° (π/2), 270° (3π/2) → reference angle 90° (π/2)
How to calculate a reference angle
The following is a step-by-step guide on how to calculate the reference angle of any angle.
First, the standard or original angle must be measured or calculated. For this example, we will assume the use of a protractor to measure an angle of 120 degrees.
Next, the quadrant that this angle lays in must be determined. The quadrant that an angle is in changes every 90 degrees. Therefore, this angle would be in the second quadrant.
Finally, the angle must be put into the formula above to calculate the reference angle. We find that the reference angle is 60 degrees.
Analyze these results and check using the graphic above to make sure it lies in the correct quadrant.
FAQ
A reference angle is the (nonnegative) angle formed between the terminal side of an angle in standard position and the x-axis. It is always between 0° and 90° (between 0 and π/2) inclusive.
For more math related calculators, click here.
